Have you ever been faced with a seemingly insurmountable mountain of numbers and units in a chemistry problem? Feeling overwhelmed by the sheer chaos of converting grams to moles or liters to milliliters? Fear not, intrepid student of chemistry! This is where the magical tool of dimensional analysis comes to your rescue. In this comprehensive guide, we’ll embark on a journey through the intricacies of Chemistry Unit 1 Worksheet 6, unraveling the mystery of dimensional analysis and empowering you to tackle complex conversions with confidence.
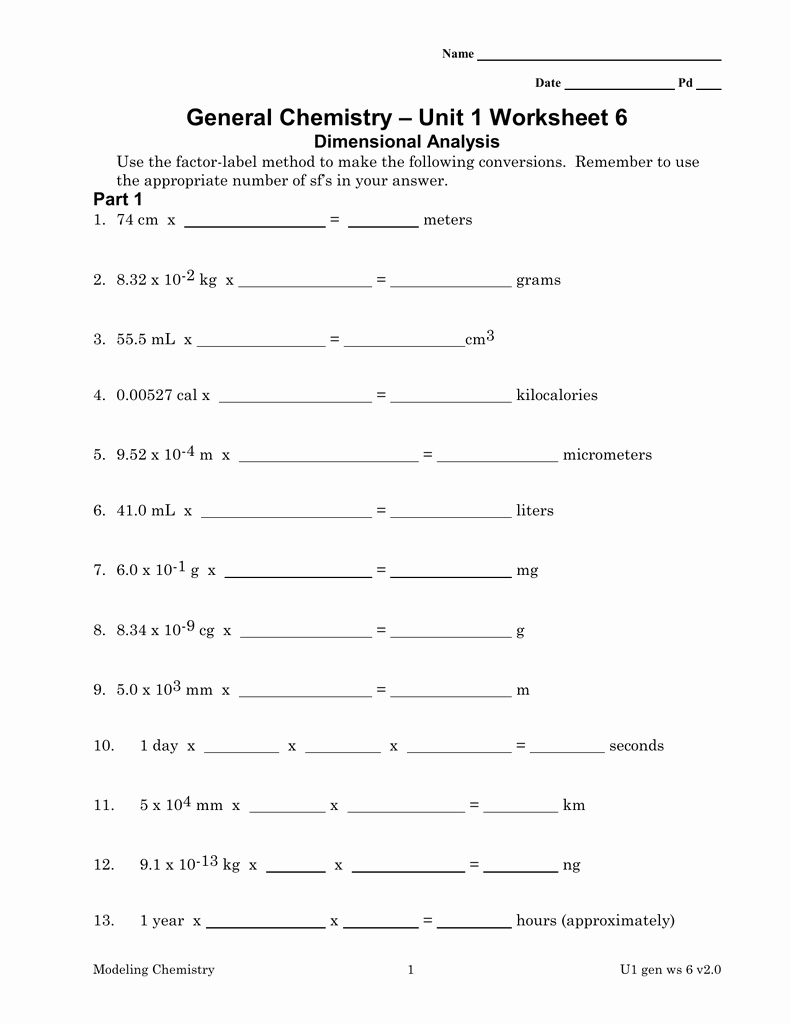
Image: chessmuseum.org
Dimensional analysis, a fundamental technique in chemistry, provides a structured framework for transforming units and solving problems, ensuring that your calculations are both accurate and logical. It’s essentially a “unit-matching” game that eliminates the guesswork, guaranteeing that you’re working with the correct units and arriving at the desired answer. Think of it as a well-organized toolbox, equipped with a collection of conversion factors that act as your trusty tools to manipulate units and solve problems.
Understanding the Fundamentals: Units and Conversion Factors
Before we delve into the mechanics of dimensional analysis, let’s first grasp the foundation: the concept of units and conversion factors. Units are the building blocks of measurement, defining the quantity of a particular physical property. For instance, “meters” measure distance, “grams” measure mass, and “seconds” measure time. Each unit corresponds to a specific magnitude, allowing us to quantify the world around us.
Conversion factors are the bridge between different units. They represent a ratio of equivalent amounts in different units, ensuring that the value of a quantity remains the same even when expressed in different ways. For example, the conversion factor for converting meters to centimeters is 1 meter = 100 centimeters. This tells us that 1 meter and 100 centimeters represent the same distance.
Mastering the Art of Dimensional Analysis: A Step-by-Step Guide
Now, let’s delve into the practical application of dimensional analysis, armed with our understanding of units and conversion factors. When tackling a problem involving units, follow these key steps to achieve a successful conversion:
1. Identify the Desired Unit and Starting Unit
Begin by clearly identifying the desired unit you want to obtain and the starting unit given in the problem. For instance, if we want to convert 5 grams to kilograms, the desired unit is kilograms and the starting unit is grams.

Image: wks.udlvirtual.edu.pe
2. Gather the Necessary Conversion Factors
Next, gather the necessary conversion factors to bridge the gap between the starting unit and the desired unit. Based on our example, we need a conversion factor relating grams and kilograms. This would be 1000 grams = 1 kilogram.
3. Set Up the Conversion as a Series of Fractions
Now, arrange the conversion factors as a series of fractions. The starting unit should be in the denominator of the first fraction, and the desired unit should appear in the numerator of the last fraction. This ensures that all unwanted units cancel out, leaving only the desired unit.
Example: Converting 5 Grams to Kilograms
- Step 1: Desired unit: kilograms. Starting unit: grams.
- Step 2: Conversion factor: 1000 grams = 1 kilogram.
- Step 3: Set up the conversion as follows:
5 grams * (1 kilogram / 1000 grams) = 0.005 kilogramsNotice how the units “grams” cancel out, leaving us with the desired unit “kilograms.” The result of the calculation is 0.005 kilograms, the correct conversion of 5 grams to kilograms.
Conquering Chemistry Unit 1 Worksheet 6: A Deep Dive into Applications
Let’s put our newfound knowledge of dimensional analysis to the test with specific examples from Chemistry Unit 1 Worksheet 6. These exercises will solidify your understanding and provide valuable practice in applying the technique.
Problem 1: Converting Moles to Grams
Imagine you’re given 2.5 moles of sodium chloride (NaCl) and asked to determine its mass in grams. Here’s how we approach this problem using dimensional analysis:
- Step 1: We need to convert moles (mol) to grams (g).
- Step 2: The necessary conversion factor is the molar mass of NaCl, which is approximately 58.44 g/mol (find this on the periodic table).
- Step 3: Set up the conversion:
2.5 mol NaCl * (58.44 g NaCl / 1 mol NaCl) = 146.1 g NaClNotice how the units “mol” cancel out, leaving us with “g,” the desired unit.
Problem 2: Converting Liters to Milliliters
Now, let’s say you have 0.75 liters of a solution and want to express this volume in milliliters.
- Step 1: We need to convert liters (L) to milliliters (mL).
- Step 2: The conversion factor is 1 L = 1000 mL.
- Step 3: Set up the conversion:
0.75 L * (1000 mL / 1 L) = 750 mLAgain, the units “L” cancel out, leaving us with “mL.”
Beyond the Basics: Advanced Dimensional Analysis Techniques
While the basic framework of dimensional analysis is straightforward, mastering its application requires practice and a keen eye for detail. Here are some advanced techniques that can enhance your problem-solving capabilities:
-
Multi-Step Conversions: When dealing with complex conversions involving multiple units, break the process into smaller steps, focusing on each intermediate unit. For example, converting cubic meters to milliliters would require multiple conversion factors linking cubic meters, cubic centimeters, and milliliters.
-
Unit Cancellations: Pay close attention to unit cancellations throughout the process. Ensure that each unwanted unit cancels out, leaving only the desired unit. This guarantees that your calculations are logically sound and accurate.
-
Multiple Conversion Factors: Utilize multiple conversion factors simultaneously when necessary. For example, converting pounds to grams might involve conversion factors linking pounds to kilograms and kilograms to grams.
The Power of Dimensional Analysis: A Catalyst for Success
Dimensional analysis is not merely a collection of calculations; it’s a powerful tool that unlocks the deeper meaning behind numbers and units. It promotes a fundamental understanding of how quantities relate to one another, paving the way for a more intuitive grasp of chemistry concepts. By mastering dimensional analysis, you’re not only solving problems but also developing a robust analytical framework that will serve you well in future scientific endeavors.
Continuing Your Journey: Exploring Beyond Unit 1
As you delve deeper into chemistry, dimensional analysis will continue to be your steadfast companion. You will encounter even more diverse and challenging problems, but armed with a thorough understanding of this technique, you’ll navigate these challenges with confidence.
- Chemistry Unit 2: Stoichiometry –dimensional analysis plays a crucial role in calculating reactant and product amounts in chemical reactions.
- Chemistry Unit 3: Solutions –dimension analysis is essential for determining solution concentrations, diluting solutions, and performing various calculations involving solutes and solvents.
- Chemistry Unit 4: Thermochemistry –dimensional analysis helps to calculate heat changes and work done in chemical reactions.
As you explore these units and beyond, remember that dimensional analysis is your secret weapon, ensuring that your calculations are accurate, reliable, and well-supported by a logical framework.
Chemistry Unit 1 Worksheet 6 Dimensional Analysis Answer Key
Empowering the Next Generation of Chemists: A Final Word
The journey of learning chemistry is an exciting odyssey, filled with challenges and triumphs. Dimensional analysis serves as a powerful guide, illuminating the path toward understanding and solving complex problems. Embrace this tool, practice its applications, and soon you’ll find yourself mastering seemingly complex concepts with ease. So, continue exploring, continue questioning, and most importantly, continue learning. The world of chemistry awaits your discovery!






