Unlocking the Mysteries of the Distance and Midpoint Formulas – A Homework 3 Solution Guide

Have you ever stared at a geometry problem involving two points and wondered how to find the distance between them or their exact midpoint? You’re not alone! These seemingly simple tasks can quickly become complex, especially when dealing with complicated coordinate systems. But fear not, dear student! This guide will equip you with the knowledge and tools needed to master the distance and midpoint formulas, turning those intimidating problems into manageable challenges.

Image: athensmutualaid.net

These formulas, while appearing abstract, are rooted in the fundamental concepts of geometry. They allow us to analyze and understand the relationships between points in space. Whether trying to determine the length of a line segment connecting two cities on a map, finding the center of a line segment representing a physical object, or even understanding the position of celestial bodies, these formulas are indispensable tools for problem-solving in various fields.

Unveiling the Secrets: Demystifying the Formulas

The Distance Formula: Measuring the Gap Between Points

Imagine two points in a coordinate plane – they could be your house and your best friend’s apartment, for example. How would you calculate the straight-line distance between them? This is where the distance formula comes in handy!

The distance formula is derived from the Pythagorean theorem. It utilizes the coordinates of the two points (x1, y1) and (x2, y2) and calculates the distance, ‘d’, between them using the following equation:

d = √[(x2 – x1)² + (y2 – y1)²]

Let’s break down this formula step by step:

1. Find the difference in the x-coordinates: Subtract the x-coordinate of the first point (x1) from the x-coordinate of the second point (x2).
2. Find the difference in the y-coordinates: Subtract the y-coordinate of the first point (y1) from the y-coordinate of the second point (y2).
3. Square both differences: Take the squares of the differences found in steps 1 and 2.
4. Sum the squared differences: Add the results obtained in step 3.
5. Take the square root: Find the square root of the sum calculated in step 4.

The result of this calculation is the exact distance between the two points, expressed in the same unit as the coordinates (e.g., meters, miles, etc.).

The Midpoint Formula: Finding the Central Point

Now, let’s imagine you’re planning a hiking trip with a friend. You know the starting point and the final destination, but you want to pinpoint a perfect spot for a lunch break – the midpoint. This is where the midpoint formula comes in!

The midpoint formula gives you the exact coordinates of the point that is precisely halfway between two other points. It is expressed as follows:

Midpoint = [(x1 + x2) / 2, (y1 + y2) / 2]

Let’s understand this formula step by step:

1. Sum the x-coordinates: Add the x-coordinate of the first point (x1) and the x-coordinate of the second point (x2).
2. Sum the y-coordinates: Add the y-coordinate of the first point (y1) and the y-coordinate of the second point (y2).
3. Divide each sum by 2: Divide the sum found in step 1 by 2 to get the x-coordinate of the midpoint, and divide the sum found in step 2 by 2 to get the y-coordinate of the midpoint.

The final result represents the coordinates of the midpoint, showing you the exact location of the point halfway between your starting and ending points.

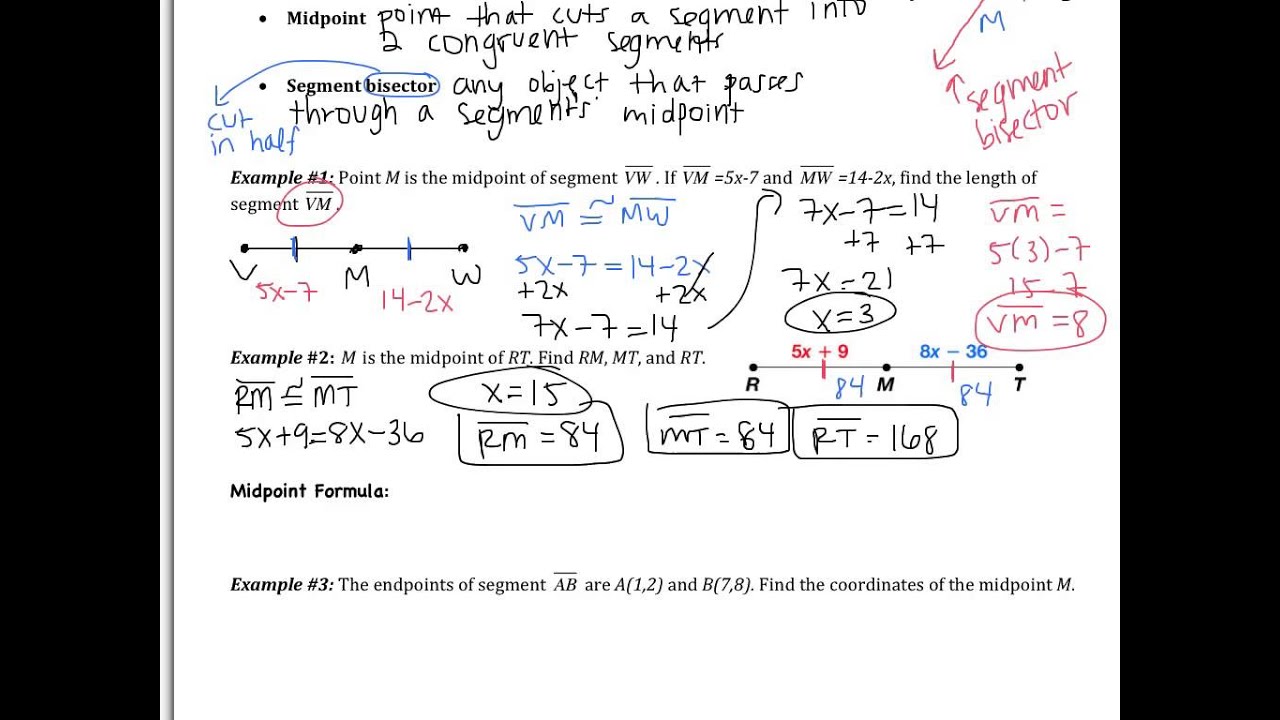
Image: www.abhayjere.com

Putting the Knowledge to Practice: Solving Real-World Problems

Now that we’ve demystified the formulas, let’s see them in action! Here are some real-world problems solved using the distance and midpoint formulas:

Scenario 1: Finding the Distance between Two Cities

Let’s say you need to travel from New York City to Los Angeles. You know the coordinates of both cities:

• New York City: (40.7128° N, 74.0060° W)
• Los Angeles: (34.0522° N, 118.2437° W)

Using the distance formula, you can calculate the straight-line distance between these cities:

1. Differences in coordinates:

   • x2 – x1 = 118.2437° W – 74.0060° W = 44.2377°
   • y2 – y1 = 34.0522° N – 40.7128° N = -6.6606°

2. Squared differences:

   • (44.2377° )² = 1955.99°²
   • (-6.6606° )² = 44.36°²

3. Sum of squared differences: 1955.99°² + 44.36°² = 2000.35°²

4. Square Root: √2000.35°² = 44.72°

Therefore, the straight-line distance between New York City and Los Angeles is approximately 44.72 degrees. This demonstrates how the distance formula can be used to measure distances between faraway places.

Scenario 2: Determining the Center of a Line Segment

Imagine you have a metal rod, and you need to find its exact center. You know the coordinates of both ends of the rod:

• Point A: (2, 5)
• Point B: (8, 1)

Using the midpoint formula, we can find the exact coordinates of the center of the rod:

1. Sum of x-coordinates: 2 + 8 = 10

2. Sum of y-coordinates: 5 + 1 = 6

3. Dividing by 2:

   • x-coordinate of midpoint = 10/2 = 5
   • y-coordinate of midpoint = 6/2 = 3

Therefore, the center of the metal rod is at the point (5, 3). This shows how the midpoint formula can be applied to find the center of any line segment or object.

Mastering the Formulas for Success

Learning the distance and midpoint formulas is not just about memorizing equations; it’s about understanding their applications and how they connect to various real-world scenarios. By practicing these formulas through diverse examples and problems, you’ll gain confidence in your ability to solve complex geometrical tasks.

Remember, these formulas are powerful tools for understanding and analyzing relationships between points in space. They are used in fields such as engineering, architecture, astronomy, and cartography, where precise measurements and calculations are crucial. By mastering these formulas, you are not only fulfilling homework assignments but also gaining valuable skills that can be applied in various aspects of life.

Homework 3 Distance And Midpoint Formulas Answer Key

Conclusion

This guide has equipped you with the necessary knowledge and insights into the distance and midpoint formulas. With practice and understanding, you can now tackle any problem involving these concepts head-on, unraveling the mysteries of distance and midpoint calculations. Remember, these formulas are essential not just for academic success but also for real-world applications. So, go forth, armed with this knowledge, and conquer those geometrical challenges with confidence!